What Is The 3-Body Problem And Why Is It So Hard To Solve?

The 3-body problem is a persistent mystery that has intrigued astrophysicists worldwide since the time of Isaac Newton and the discovery of gravity. It is a hypothetical concept that describes the motion of three bodies under their mutual gravitational interactions, but this is an extremely challenging task due to the unpredictable nature of the system.

This complex nature of the system raises questions like, what is the 3-body problem, why is it so hard to solve, and the feasibility of scenarios depicted in the Netflix series “The three-body Problem”.

When the two moving bodies move about their axes in a circular path, they come back to their starting positions after some time. But when a third body is added to them things become more complex. The third body attracts the two bodies disrupting their paths.

It is straightforward to understand that the Earth orbits the sun due to the sun’s stronger gravitational pull because the stronger gravitational forces of the sun dominate over the weaker forces of each planet in the solar system. Similarly, the moon revolves around the Earth because it is within Earth’s gravitational influence.

The essence of the 3-body problem is: how to predict and stabilize the orbits of 3 interacting bodies. The third object’s presence causes wild movements, potentially sending the interacting bodies into collisions or chaotic orbits.

What is the two-body problem?

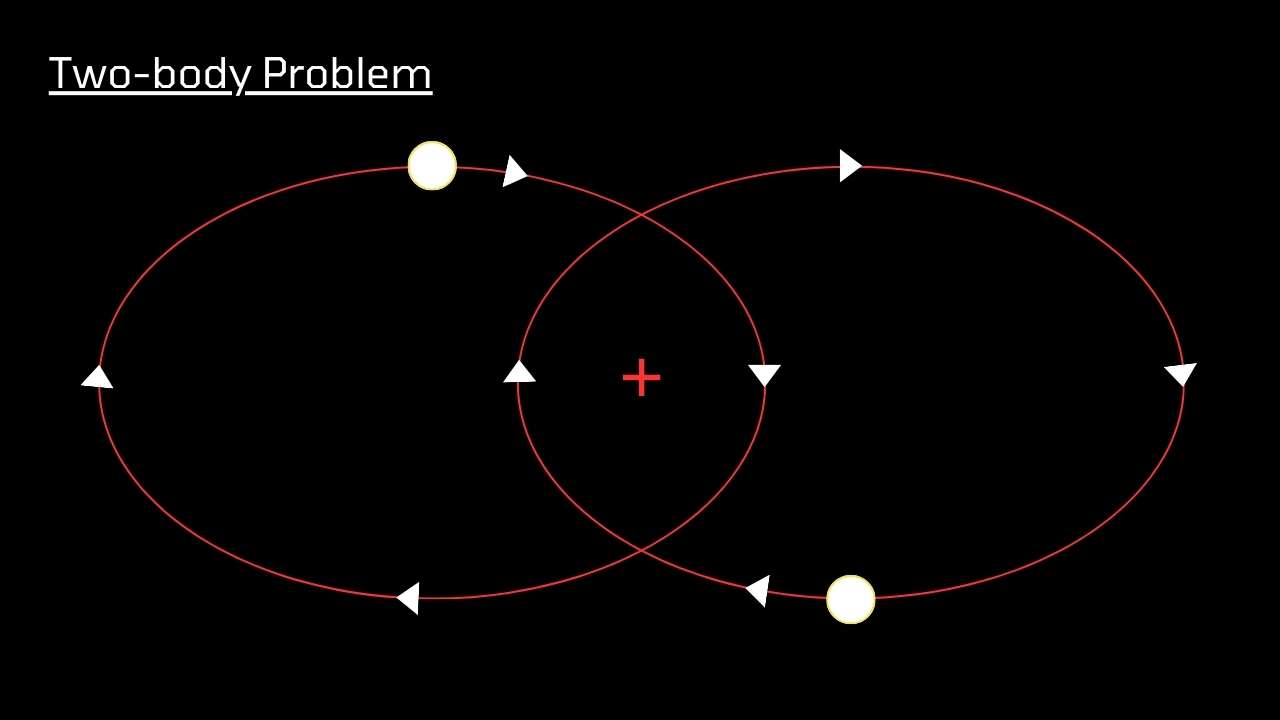
In 1687, Newton worked on a concept, known as the “two-body problem”. According to it the two objects whose gravitational fields interact will form stable and predictable orbits with unchangeable paths. This principle is also applied to the binary star systems in which 2 stars revolve around a common centre of mass (COM). Stable planetary orbits are also included in binary star systems.

Also Read: If time is an illusion, why do we age?

However, when a third body is added to the two-body system, it creates complexity and instability instead of stabilizing it. To understand this concept, Dr. Charles J. Horowitz said the key here is the Law of conservation of energy.

The energy in a closed system can neither be created nor be destroyed.

Two stars can exchange energy and possibly eject an orbiting planet.

Motion Of Three Bodies:

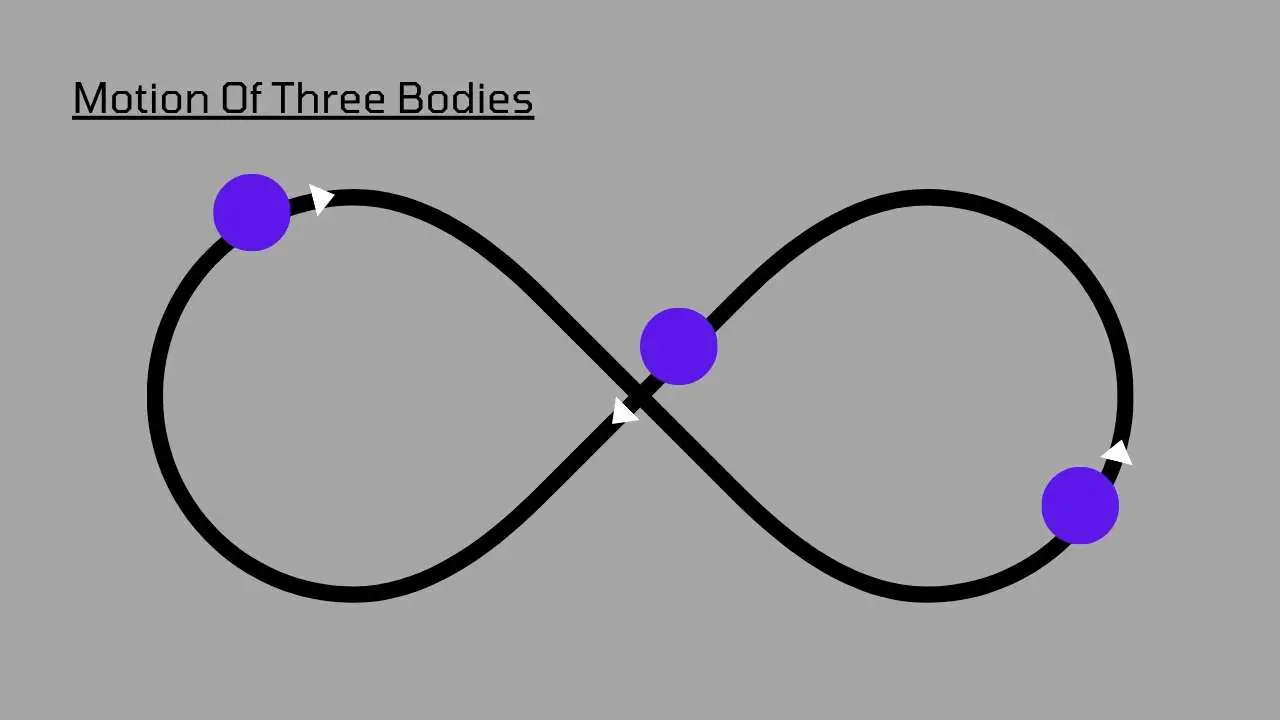
The motion of bodies is dependent on their initial states, their positions, velocities, and masses. When one of these variables undergoes a change, the results might be different.

Shane Ross, an applied mathematician at VirginiaTech, told Live Science:

With one small change, you can either fall to the right or you could fall to the left.

Position:

When the velocity of each body changes, their positions also change accordingly.

Velocity:

The bodies exert gravitational force on each other. So, the velocity, speed, and direction of each body change due to these gravitational forces. For instance, a game of tug of war changing all its directions continuously. You and your friend are having a rope and pull on each other, the direction and speed can change how the rope is pulled by others.

Mass:

Mass influences gravitational pull. It determines how strong the gravitational pull is on the other two bodies. The more massive the body is, the more is the gravitational pull. Likewise, in the game of tug of war, the rope would be pulled harder by the more massive body.

Law of conservation of energy:

The 3-body problem focuses on three moving bodies that interact with each other through gravity. This system includes kinetic energy, potential energy, and the energy of the interaction of bodies.

Kinetic energy is transformed to potential as the bodies come closer or apart from each other. However, the potential energy is transformed into kinetic energy as the body accelerates or decelerates. While total energy remains constant. Since the 3-body system is a closed system with no external force or energy. When the bodies move, the energy is converted from one form to another i.e. potential to kinetic and vice versa; but is always conserved.

Why is the Law of conservation of energy applied to the 3-body problem?

• For predicting the motion of interacting bodies timely.
• For a better understanding of the dynamics of the system.
• For accurate calculations.

Why is the 3-body problem so hard to solve?

There are some features of the 3-body problem that combine to make it a complex challenge for scientists.

Inconsistent behavior

This system has a very unpredictable nature. Thus, if small changes occur in the initial conditions, the outcomes that markedly differ over time will be observed. This chaos makes long-term prediction nearly impossible because it requires precise initial data.

Exchange of energy

In a 3-body system, the bodies continuously interchange energy and angular momentum. Due to these energy exchanges, complex changes in the orbits of these interacting bodies occur. Hence, long-term behavior prediction becomes more difficult.

Complexity of computation

The 3-body system behavior can be represented by numerical calculations, but it needs an enormous amount of processing power and can only give precise solutions over finite time intervals.

Lack of symmetry

Because of the symmetry in the two-body problem, mathematics becomes easier. But this symmetry is lost in the 3-body system, leading to complex interactions that are hard to explain.

Nonlinear dynamics

There are nonlinear equations of motion in the 3-body system. Hence slight changes in the initial conditions can result in different outcomes and make this system very unpredictable and sensitive.

Historical solutions of the 3-body problem

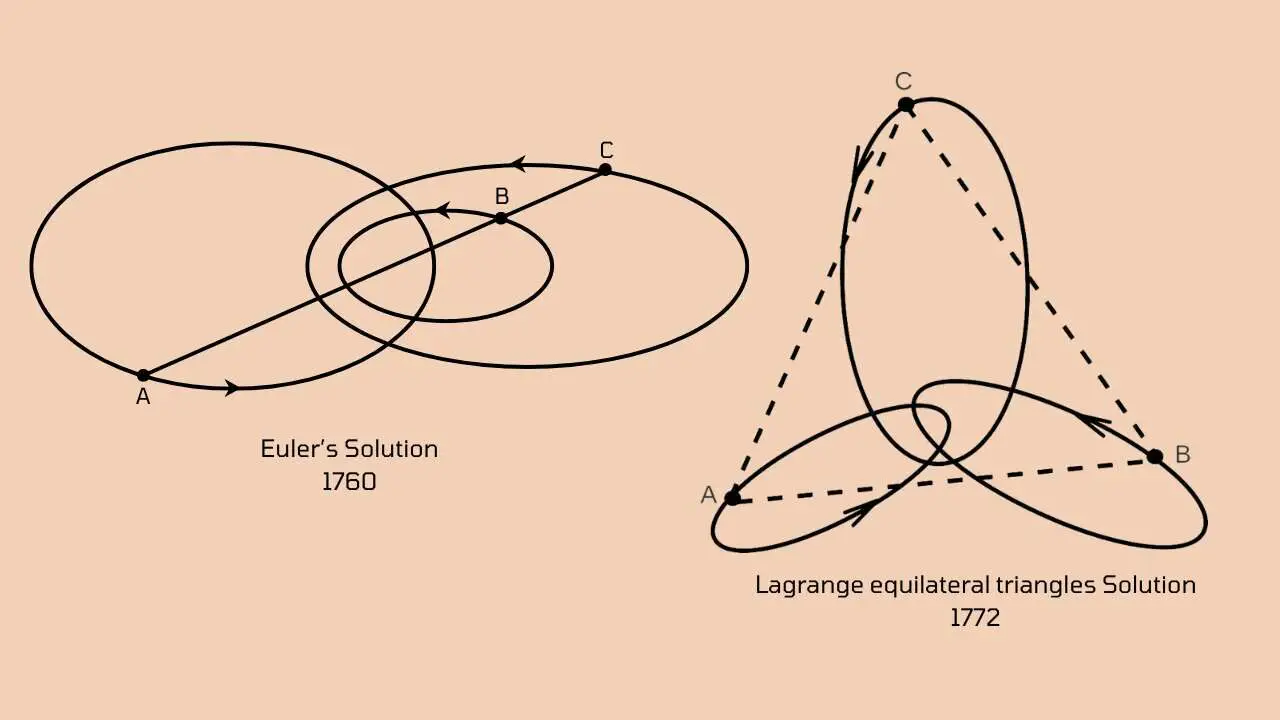
In 1760, Euler made contributions to the 3-body problem. According to Euler;

The parameter that controls the relative distances among three collinear bodies is given by a quintic equation.

Actually, the 3-body problem is to solve for the motion of a body which is acted upon by the gravitational field of the other two bodies.

In 1772, Lagrange dealt with the same problem and demonstrated that there are two pattern solutions for any three masses having circular orbits. The two main constant patterns are given below:

• One where the three bodies remain collinear
• Other where the bodies occupy the vertices of  two equilateral triangles

The Lagrange points also called liberation points and L-points are the points close to two large orbiting bodies. The two objects exert unbalanced gravitational force at a point resulting in the alternation of orbit of whatever is at that point. At Lagrange’s points, the centrifugal force and gravitational force of two large bodies balance each other. This shows that Lagrange’s points are the best to locate the satellites.

Euler’s Quintic equation:

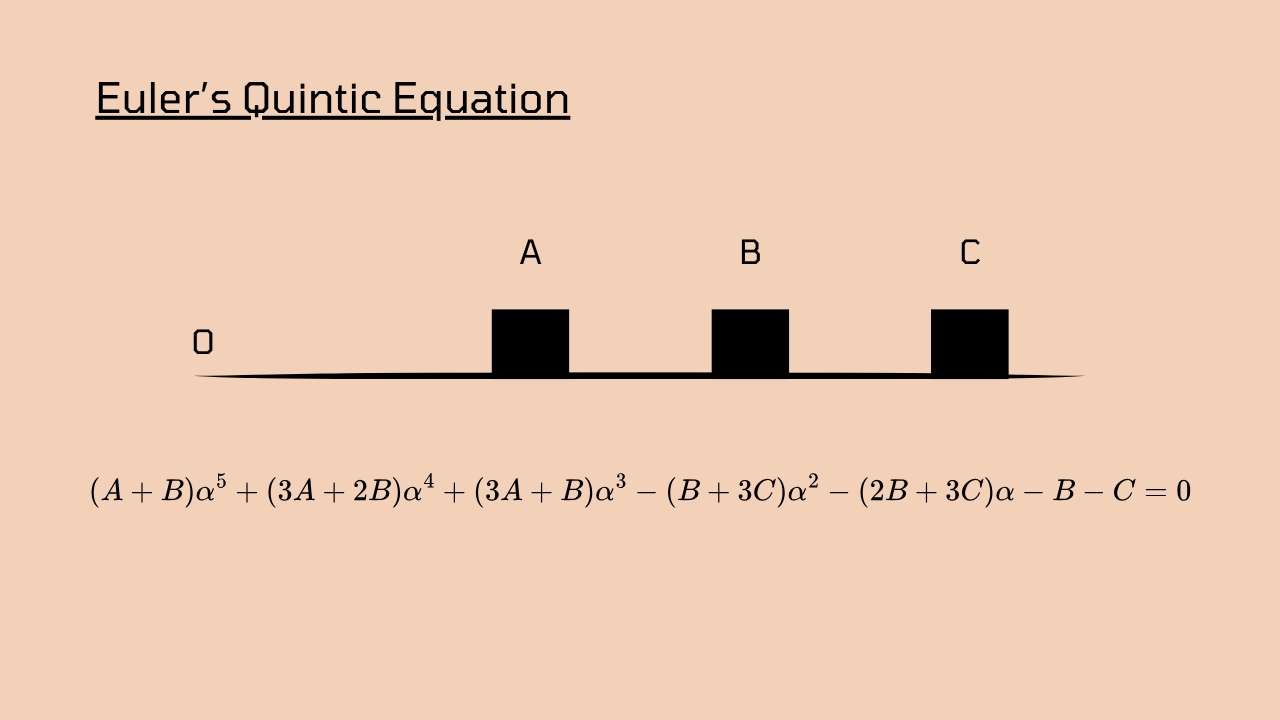
Euler considers three bodies lying on a straight line attract each other by their central forces which are inversely proportional to the square of their separation distance. This is called inverse square law. The most significant contribution of this publication was to explain that there is a parameter called alpha. Alpha controls the relative distances among the three bodies. The quintic equation is as follows:

(𝐴 + 𝐵) ∝ 5+ (3𝐴 + 2𝐵) ∝ 4+ (3𝐴 + 𝐵) ∝ 3− (𝐵 + 3𝐶) ∝ 2− (2𝐵 + 3𝐶) ∝ −𝐵 − 𝐶 = 0

It does not only show the relative distances among the three bodies but also the formulation considering the four aligned bodies.